MIC3231YTSE【PDF 14/19 页】IC LED DRVR HP CONS CURR 16TSSOP

收藏本站

您好，
买卖IC网欢迎您。
请登录
免费注册

买卖IC网

Sheet目录343

10页 11页 12页 13页 [14页]15页 16页 17页 18页

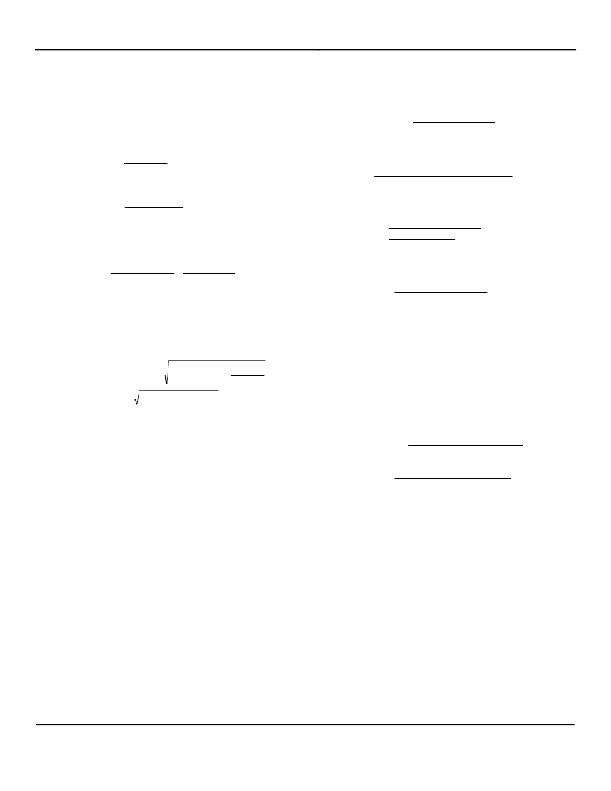
0 . 45 = I RAMP × R SLC × D + I L _ pk Limit × R CS

V OUT ? V IN MIN ) × R CS

R SLC =

Eq. (13)

L = IN

= 43 μ H

12 V × 0 . 56 × 2 μ s

R CS =

Eq. (13a)

I in _ PP =

V IN _ nom × D nom × T 12 v × 0 . 56 × 2 us

R CS =

( 28 v ? 8 v ) × ( 0 . 50 ) + 1 . 9 A = 179 m Ω

( 28 ? 8 ) × 150 m Ω

R SLC = = 511 Ω

( I IN _ RMS _ max ) 2 ? ( I IN 12 PP )

Eq. (13b)

I IN _ AVE _ max =

Micrel, Inc.

(see the current waveforms in Figure 5).

It can be difficult to find large inductor values with high

saturation currents in a surface mount package. Due to

this, the percentage of the ripple current may be limited

by the available inductor. It is recommended to operate

in the continuous conduction mode. The selection of L

described here is for continuous conduction mode.

V × D × T

I in _ PP

Using the nominal values, we get:

L =

0 . 31 A

Select the next higher standard inductor value of 47μH.

Going back and calculating the actual ripple current

gives:

= = 0 . 29 A PP

L 47 uh

The average input current is different than the RMS input

current because of the ripple current. If the ripple current

is low, then the average input current nearly equals the

RMS input current. In the case where the average input

current is different than the RMS, Equation 10 shows the

following:

MIC3230/1/2

Eq. (14a)

To calculate the value of the slope compensation resistance,

R SLC , we can use Equation 5:

MAX

L × 250 μ A × F SW

First we must calculate R CS , which is given below in

Equation 15:

Eq. (15) 0 . 45

( VOUT MAX ? VIN MIN ) × D max + I L _ pk Limit

L × F SW

Therefore;

0 . 45

47 μ H × 500 kHz

Using a standard value 150m ? resistor for R CS , we obtain

the following for R SLC :

47 μ H × 250 μ A × 500 kHz

Use the next higher standard value if this not a standard

value. In this example 511 ? is a standard value.

Check: Because we must use a standard value for Rcs and

R SLC; I L _ pk Limit may be set at a different level (if the calculated

value isn’t a standard value) and we must calculate the

actual I L _ pk Limit value (remember I L _ pk Limit is the same as

I IN _ AVE _ max =

( 1 . 64 ) 2 ? ( 0 . 29 ) 2 / 12 ≈ 1 . 64 A

I in _ pk Limit ).

I in _ pk Limit =

I in _ actual Limit =

= 2 . 34 A

Eq. (13c) P INDUCTOR = I in _ RMS _ max × DCR

The Maximum Peak input current I L_PK can found using

equation 11:

I L _ PK _ max = I IN _ AVE _ max + 0 . 5 × I L _ PP _ max = 1 . 78 A

The saturation current (I SAT ) at the highest operating

temperature of the inductor must be rated higher than

this.

The power dissipated in the inductor is:

Current Limit and Slope Compensation

Having calculated the I L_pk above, We can set the current

limit 20% above this maximum value:

I L _ pk Limit = 1 . 2 × 1 . 6 A = 1 . 9 A

The internal current limit comparator reference is set at

0.45V, therefore when V IS _ PIN = 0 . 45 , the IC enters

current limit.

Eq. (14) 0 . 45 = ( V A PK + Vcs PK )

Where V A PK is the peak of the V A waveform and

Vcs PK is the peak of the Vcs waveform

Rearranging Equation 14a to solve for I L _ pk Limit :

( 0 .45 ? I RAMP × R SLC × D )

R CS

( 0 . 45 ? 250 ua × 511 × 0 . 75 )

. 150

This is higher than the initial 1 . 2 × I L _ PK _ max = 1 . 9 A limit

because we have to use standard values for R CS and for

R SLC . If I in _ actual Limit is too high than use a higher value for

R CS . The calculated value of R CS for a 1.9A current limit was

179m ? . In this example, we have chosen a lower value

which results in a higher current limit. If we use a higher

standard value the current limit will have a lower value. The

designer does not have the same choices for small valued

resistors as with larger valued resistors. The choices differ

from resistor manufacturers. If too large a current sense

resistor is selected, the maximum output power may not be

able to be achieved at low input line voltage levels. Make

sure the inductor will not saturate at the actual current limit

I in _ actual Limit .

Perform a check at I IN =2.34Apk.

V IS _ PIN = 250 μ A × ( 0 . 78 ) × 511 Ω + 2 . 34 A × 150 m Ω = 0 . 45 V

March 2011

M9999-030311-D

发布紧急采购，3分钟左右您将得到回复。

采购需求

(若只采购一条型号，填写一行即可)

*型号	*数量	厂商	批号	封装

添加更多采购

采购需求

发布成功！您可以继续发布采购。也可以进入我的后台，查看报价

发布成功！您可以继续发布采购。也可以进入我的后台，查看报价

我的联系方式